WEB TUTORIAL - Group Theory

[Re₂Cl₈]^2- Worked Example

D4h	E	2C4 (z)	C2	2C'2	2C''2	i	2S4	h	2v	2d	Linear Functions, Rotations	Quadratic Functions	Cubic Functions
A1g	+1	+1	+1	+1	+1	+1	+1	+1	+1	+1	-	x2+y2, z2	-
A2g	+1	+1	+1	-1	-1	+1	+1	+1	-1	-1	Rz	-	-
B1g	+1	-1	+1	+1	-1	+1	-1	+1	+1	-1	-	x2-y2	-
B2g	+1	-1	+1	-1	+1	+1	-1	+1	-1	+1	-	xy	-
Eg	+2	0	-2	0	0	+2	0	-2	0	0	(Rx, Ry)	(xz, yz)	-
A1u	+1	+1	+1	+1	+1	-1	-1	-1	-1	-1	-	-	-
A2u	+1	+1	+1	-1	-1	-1	-1	-1	+1	+1	z	-	z3, z(x2+y2)
B1u	+1	-1	+1	+1	-1	-1	+1	-1	-1	+1	-	-	xyz
B2u	+1	-1	+1	-1	+1	-1	+1	-1	+1	-1	-	-	z(x2-y2)
Eu	+2	0	-2	0	0	-2	0	+2	0	0	(x, y)	-	(xz2, yz2) (xy2, x2y), (x3, y3)

h = 16

Now we have all of the required parts we simply put then together into the Reduction Formula one row at a time. This calculation will be quite long, but it is important that you are able to compute a reduction of this size.

It is worth doing this on a piece of paper rather than trying in your head before checking your answers!

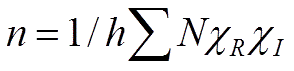
The calculation has not been implicitly shown here For a recap of how to use the Reduction Formula please click HERE:

The result you should have obtained for each row is as follows:

A_1g = 32/16 = 2
A_2g = 0/16 = 0
B_1g = 16/16 = 1
B_2g = 0/16 = 0
E_g = 16/16 = 1
A_1u = 0/16 = 0
A_2u = 16/16 = 1
B_1u = 0/16 = 0
B_2u = 16/16 = 1
E_u = 16/16 = 1

So there are two A_1g, one B_1g, one E_g, one A_2u, one B_2u and one E_u representations.

So what does this mean? ⇒